Geometric Progression Sum to Infinity
The formula works for any real numbers a and r except. In Maths NCERT Solutions Class 10 Chapter 5 students will learn about the arithmetic progression.

Mathwords Infinite Geometric Series
The sum of the distance travelled during a drivers perceptionreaction time and the distance travelled thereafter while braking to a stop.
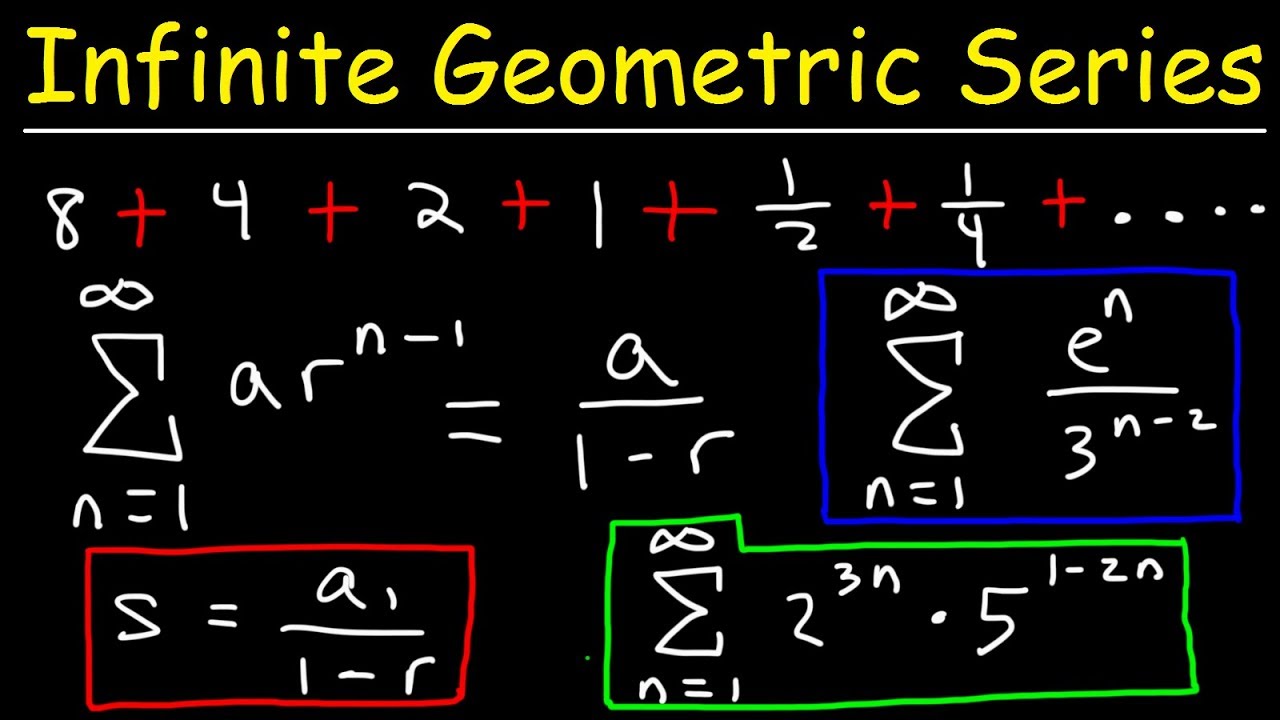
. How do we check whether a series is an arithmetic progression or not. Therefore to calculate series sum one needs somehow to find the expression of the partial series sum S nIn our case the series is the decreasing geometric progression with ratio 13. Letting a be the first term here 2 n be the number of terms here 4 and r be the constant that each term is multiplied by to get the next term here 5 the sum is given by.
Each successive term is obtained in a geometric progression by multiplying the common ratio to its preceding term. N th term for the GP. A POLICY on GEOMETRIC DESIGN of HIGHWAYS and STREETS 2001 American Association of State Highway and Transportation Officials.
For example the series is geometric because each successive term can be obtained by multiplying the previous term by In general a geometric series is written as where is the coefficient of each term and is the. Consider the GP a ar ar 2ar n-1. But be careful.
Derivation of Sum of GP. The Product of all the numbers present in the geometric progression gives us the overall product. A n ar n-1.
If n consecutive natural numbers. Thus the explicit formula is. So our infnite geometric series has a finite sum when the ratio is less than 1.
What all will you get under EduRev Infinity Package for CAT. Download Free PDF View PDF. In this section we will learn to find the sum of geometric series.
Sum to infinity of the series 3 2. The sum of the infinite series 1 2. The amount of cross-slope provided on a curve to help counterbalance.
The formula for the n th term of an AP. . R_1 r_2 cdots r_n -1n fraca_0.
Geometric Progression Sum Of Gp. The family of natural numbers includes all the counting numbers starting from 1 till infinity. Mean or average of the set of numbers which is computed by adding all the terms in the set of numbers and dividing the sum by a total number of terms.
Practice more questions. Its geometric mean is zero and the harmonic mean is infinity. Every answer is written according to the.
8 terms of 3 3 3 3. Doc 6 pages. As in the quadratic case Vietas formula gives an equation to find the sum of roots.
Grade 10 Science Module 1st Quarter Luwen Borigas. The NCERT Solutions for Class 10 Maths Chapter 5 PDF file available for free can help students to score good marks. SANRAL Geometric Design Guide - Free ebook download as PDF File pdf Text File txt or read book online for free.
If r 1 r 1 r 1 then the sum to infinity is given by. This file is prepared by the best academic experts in India. It is very useful while calculating the Geometric mean of the entire.
Nth Term of a GP. A Policy on Geometric Design of Highways and Streets 2018 7th Edition contents. S n b 1 q n 1 q 1.
Sum Of N Terms. Students can download this PDF file by visiting Vedantu. 64 16 4 1.
In geometric progressions where r 1 in other words where r is less than 1 and greater than 1 the sum of the sequence as n tends to infinity approaches a value. Since we know in a GP the common ratio between the successive terms is constant so we will consider a geometric series of finite terms to derive the formula to find the sum of Geometric Progression. For Infinite Geometric Series.
The sum to infinity of a geometric progression. Sum to Infinite GP - Algebra Quantitative Reasoning Video. The arithmetic and geometric progression Maija Liepa.
N will tend to Infinity n Putting this in the generalized formula. The formula for the nth term of a geometric progression whose first term is a and common ratio is r is a n ar n1. I 1 n r i a n 1 a n.
And r should not be 0 because the sequence a00 is not geometric. In mathematics a geometric series is the sum of an infinite number of terms that have a constant ratio between successive terms. If a is the initial term and d is a common difference.
In the example above this gives. There are various formulae and techniques for the calculation of the sum of squares. Where b 1 - is the first element of the geometric series in our case it.
4 4 4 4 B. Exponential Sum Formulas. Problems based on Sum to n Terms of a GP.
120 days study plan to prepare for CAT with EduRev Doc. Sum to n Terms of a GP. The formula for the sum of n terms of AP.
The exponential function EXP x is defined to be the sum of the following infinite series. Write a program that reads in a REAL value and computes EXP of that value using the. The sum of the infinite GP formula is given as S n a1r where r.
Sum of the first n terms S n. R 1 r 2 r n 1 n a 0 a n. In arithmetic we often come across the sum of n natural numbers.
Sum_i1n r_i - fraca_n-1a_n. Similarly we have the following equation for the product of roots. Find the sum to infinity of each geometric sequence if it exists.
We can use this formula. A geometric progression is a sequence of numbers in which any two consecutive terms of the sequence have a common ratio. Product of the Geometric series.
It is known that the sum of the first n elements of geometric progression can be calculated by the formula. The sum of the first n terms of the AP series. 11X1 T10 03 arithmetic geometric means.
Arithmetic Progression Geometric Progression Video 0256 min. An arithmetic-geometric progression AGP is a progression in which each term can be represented as the product of the terms of an arithmetic progressions AP and a geometric progressions GP. Geometric Sequences and Sums Sequence.
A Sequence is a set of things usually numbers that are in order. In other words if you keep adding together the terms of the sequence forever you will get a finite value. Geometric series Jhon Paul Lagumbay.
So what happens when n goes to infinity. I 1 n r i a n a n 1. A geometric series is the sum of the numbers in a geometric progression.
Similarly By looking at the Real and Imaginary Parts of these Formulas sums involving sines and cosines can be obtained.
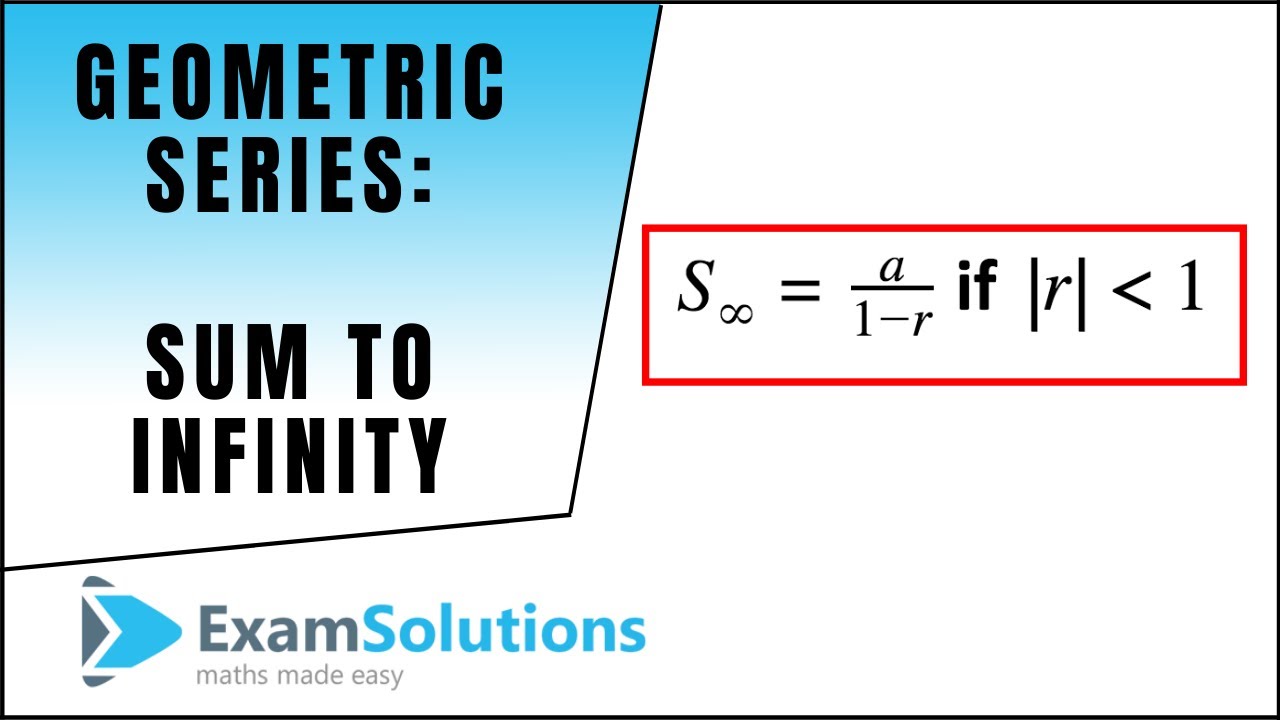
Geometric Series Sum To Infinity Examsolutions Youtube

Geometrical Progression Sum Of Infinite Terms Derivation Youtube
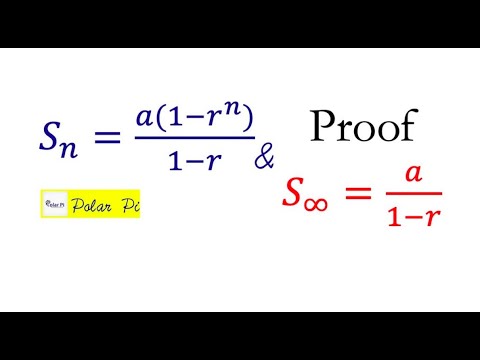
Proof Of The Geometric Series Formula Finite Infinite Youtube

Finding The Sum Of An Infinite Geometric Series Youtube
0 Response to "Geometric Progression Sum to Infinity"
Post a Comment